Difference between revisions of "The Modulus and the Conjugate of a Complex Number"
Jump to navigation
Jump to search
Ramamurthy S (talk | contribs) |
Ramamurthy S (talk | contribs) |
||
| Line 18: | Line 18: | ||
<math>=\frac{(48-36i+20i-15i^2)}{(16-9i^2)}</math><math>=\frac{(48-16i-15(-1))}{(16-9(-1))}</math><math>=\frac{63-16i}{25}</math><math>=\frac{63}{25}-\frac{16i}{25}</math> | <math>=\frac{(48-36i+20i-15i^2)}{(16-9i^2)}</math><math>=\frac{(48-16i-15(-1))}{(16-9(-1))}</math><math>=\frac{63-16i}{25}</math><math>=\frac{63}{25}-\frac{16i}{25}</math> | ||
Answer: The conjugate of <math>\frac{(3-2i)(2+3i)}{(1+2i)(2-i)}</math><math>=\frac{63}{25}+\frac{16i}{25}</math> | '''Answer''': The conjugate of <math>\frac{(3-2i)(2+3i)}{(1+2i)(2-i)}</math><math>=\frac{63}{25}+\frac{16i}{25}</math> | ||
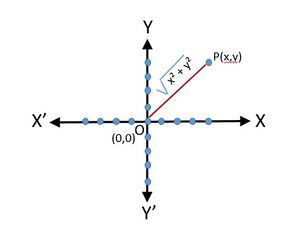
== Modulus of a Complex Number == | == Modulus of a Complex Number == | ||
[[File:Modulus-complex number.jpg|alt=Modulus of a complex number|thumb|Fig 2 - Modulus of a complex number]] | [[File:Modulus-complex number.jpg|alt=Modulus of a complex number|thumb|Fig 2 - Modulus of a complex number]] | ||
Revision as of 13:19, 11 November 2023
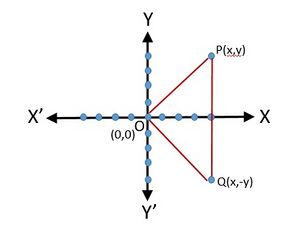
The modulus of a complex number gives the distance of the complex number from the origin in the Argand plane, whereas the conjugate of a complex number gives the reflection of the complex number about the real axis in the Argand plane.
Conjugate of a Complex Number
The representation of a complex number and its conjugate in the Argand plane are, respectively , the points and
Geometrically , the point is the mirror image of the point on the real axis (Fig 1)
Example: Find the conjugate of
Answer: The conjugate of